Understanding Horizontal Asymptotes: A Comprehensive Guide
When embarking on the journey of understanding the behavior of functions as they extend towards infinity, horizontal asymptotes stand out as a fundamental concept in calculus. This comprehensive guide aims to demystify the process of finding horizontal asymptotes, providing a deep dive into their theoretical background, practical calculation methods, and the significance they hold in mathematical analysis. By the end of this article, you will have a solid understanding of horizontal asymptotes, equipped with the knowledge to identify them across various functions.
Theoretical Foundation
Horizontal asymptotes are straight lines that a graph approaches but never actually reaches as the input values grow infinitely large or small. These asymptotes provide insight into the end behavior of functions, offering a glimpse into the function’s value as it extends towards positive or negative infinity. Unlike vertical asymptotes, which are concerned with the function’s behavior near certain values of x, horizontal asymptotes focus on the y-value that the function approaches in the limit.
Understanding Limits
At the heart of finding horizontal asymptotes is the concept of limits. A limit describes the value that a function approaches as the input (or x-value) approaches a certain point. In the context of horizontal asymptotes, we are particularly interested in the limits of a function as x approaches infinity (positive or negative). It is this foundational understanding of limits that allows mathematicians to analyze and predict the behavior of functions over vast scales.
Practical Calculation Methods
Calculating horizontal asymptotes varies depending on the type of function you’re dealing with. Here, we’ll cover the most common types: rational functions, polynomial functions, and exponential functions.
Rational Functions
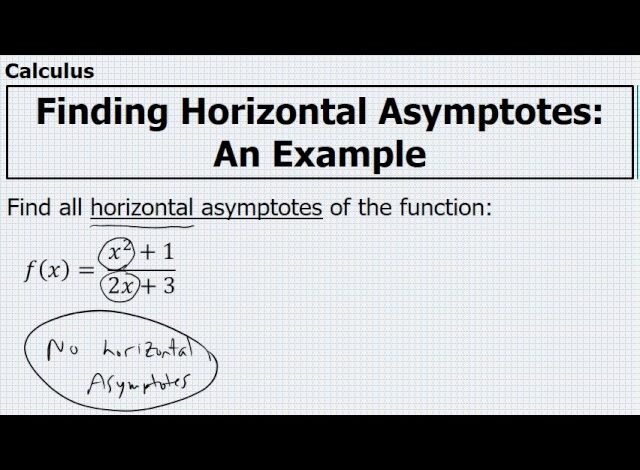
Rational functions are ratios of two polynomials. The method to find horizontal asymptotes in rational functions depends on the degrees of the numerator (N) and denominator (D) polynomials:
- If N < D, the horizontal asymptote is the x-axis, or y = 0.
- If N = D, the horizontal asymptote is the ratio of the leading coefficients of the numerator and denominator.
- If N > D, there is no horizontal asymptote (though there may be an oblique asymptote).
Polynomial Functions
Polynomial functions are simpler in terms of horizontal asymptotes—they do not have them, as the degree of the polynomial dictates that as x approaches infinity, the function itself will either approach infinity or negative infinity, based on the leading term.
Exponential Functions
Exponential functions, which have the form �(�)=��f(x)=ax, where�
a is a positive constant, behave differently. Their horizontal asymptotes depend on the base of the exponential function and the direction in which x is moving towards infinity. Typically, if the base is greater than 1, the function grows without bound, and if it is between 0 and 1, the function approaches zero, suggesting a horizontal asymptote at y = 0.
Significance in Mathematical Analysis
Horizontal asymptotes are not just academic; they have practical applications in various fields such as physics, engineering, and economics. They help in understanding the limiting behavior of functions, which is essential in modeling real-world phenomena where certain variables grow large. For example, in economics, understanding the horizontal asymptote of a cost function can provide insight into the long-term average cost of production as the scale of production increases indefinitely.
Conclusion
The concept of horizontal asymptotes is a cornerstone of calculus, providing critical insight into the behavior of functions as they stretch towards the bounds of infinity. Whether dealing with rational, polynomial, or exponential functions, the methodology to determine these asymptotes hinges on a clear understanding of limits and the fundamental properties of the functions in question. Recognizing and calculating horizontal asymptotes not only enriches our theoretical understanding of mathematical concepts but also enhances our ability to apply these concepts in analyzing the complex behaviors of real-world phenomena. As we have seen, the journey from theoretical foundation to practical application underscores the importance of horizontal asymptotes in both academic and practical contexts, making it a vital skill for students and professionals alike.




